The Diamond Theorem
(4×4 Case)
by Steven H. Cullinane
|

|
We regard
the four-diamond figure D at left as a 4×4 array of two-color
diagonally-divided square tiles. |
Let G be the group of 322,560
permutations of these 16 tiles generated
by arbitrarily mixing
random
permutations of rows and of columns with
permutations of the four 2×2 quadrants.
THEOREM: Every G-image of D (as at right, below) has some ordinary or
color-interchange symmetry.
Example:
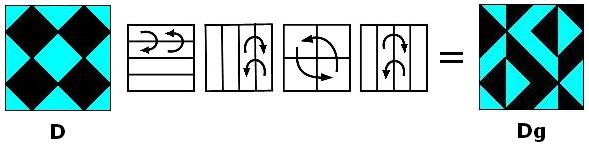
where g, a permutation in G, is a product of two disjoint 7-cycles. Note that Dg has
rotational color-interchange symmetry like that of the famed yin-yang
symbol.
Remarks:
G is isomorphic to the affine group A on the linear 4-space
over GF(2). The 35 structures of the 840 = 35 x 24 G-images of D are
isomorphic to the 35 lines in the 3-dimensional projective space over
GF(2).
This can be seen by viewing the 35 structures as three-sets of line diagrams,
based on the three partitions of the four-set of square two-color tiles
into two two-sets, and indicating the locations of these two-sets of
tiles within the 4×4 patterns. The lines of the line diagrams may be
added in a binary fashion (i.e., 1+1=0). Each three-set of line diagrams
sums to zero — i.e., each diagram in a three-set is the binary sum of the
other two diagrams in the set. Thus, the 35 three-sets of line diagrams
correspond to the 35 three-point lines of the finite
projective 3-space PG(3,2).
For example, here are the line diagrams
for the figures above:
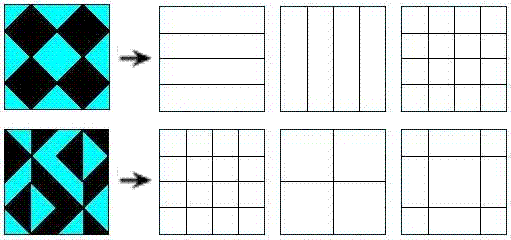
Shown below are the 15 possible line diagrams
resulting from row/column/quadrant permutations.
These 15 diagrams may, as noted above, be regarded
as the 15 points of the projective 3-space PG(3,2).

The symmetry of the line diagrams accounts for the symmetry
of the two-color patterns.
(A proof shows that a 2nx2n two-color triangular half-squares pattern
with such line diagrams must have a 2×2 center with a symmetry, and
that this symmetry must be shared by the entire pattern.)
Among the 35 structures of the
840 4×4 arrays of tiles, orthogonality (in the sense of Latin-square
orthogonality) corresponds to skewness of lines in the finite
projective space PG(3,2).
This was stated by the author in a 1978 note. (The note apparently had little effect.
A quarter-century later, P. Govaerts, D. Jungnickel, L. Storme, and J.
A. Thas wrote that skew (i.e., nonintersecting) lines in a projective space seem “at first sight not at all related” to orthogonal Latin squares.)
We can define sums and products so that the G-images of D generate an ideal
(1024 patterns characterized by all horizontal or vertical “cuts” being
uninterrupted) of a ring of 4096 symmetric patterns. There is an infinite
family of such “diamond” rings, isomorphic to rings of matrices over
GF(4).
The proof uses a decomposition technique for functions into a finite field that might be of more general use.
The underlying geometry
of the 4×4 patterns is closely related to the Miracle Octad Generator (pdf)
of R. T. Curtis– used in the construction of the Steiner system
S(5,8,24)— and hence is also related to the Leech lattice, which, as Walter Feit has remarked, “is a blown up version of S(5,8,24).”
For a movable JavaScript version of these 4×4 patterns, see The Diamond 16 Puzzle.
|
The above is an expanded version of Abstract 79T-A37, “Symmetry invariance
in a diamond ring,” by S. H. Cullinane,
Notices of the American Mathematical Society, February 1979,
pages A-193,194.
|
For a discussion of other cases of the theorem, click here.
Posted Sept. 22, 2005; replaces previous post.













